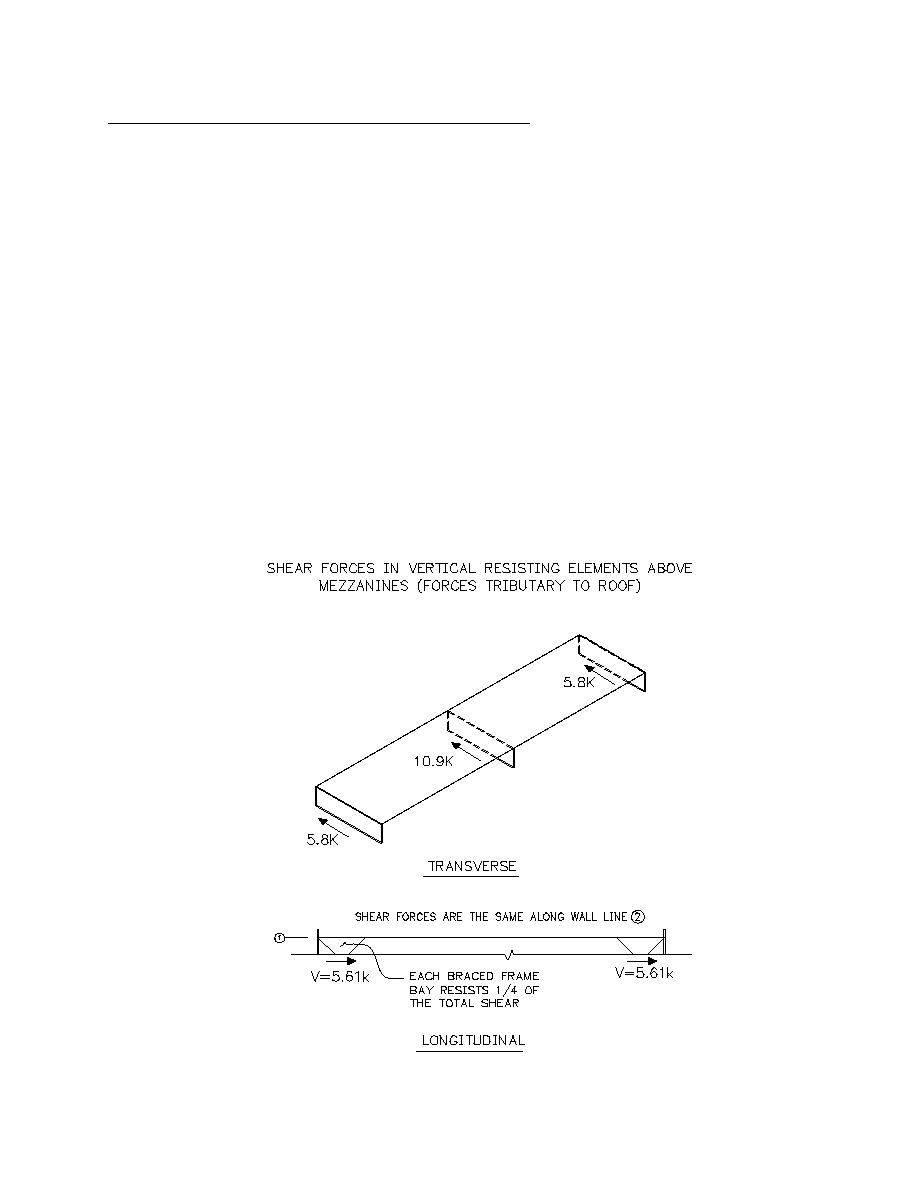
Determine shear in vertical elements due to self-weight inertial effects
The vertical resisting elements must resist the shear force transferred to them by the diaphragm in addition
to the shear forces generated by parallel walls and self-weight.
Transverse:
Typical exterior wall (A1-A2 & I1-I2)
Shear = Cs x wall weight trib to upper roof = (0.12)(14 kips) = 1.68 kips
This shear is now added to the diaphragm shear transferred to the wall;
Total shear in exterior wall above mezzanine = (1.68k + 4.08k) = 5.8 kips (25.8 KN)
Interior CMU wall E1-E2
Shear = (0.12)(22.8 kips) = 2.74 kips
Total Shear = (2.74 k + 8.16 k) = 10.9 kips (48.48 KN)
Longitudinal:
The inertial forces of the metal panel walls and roll-up doors are collected by the edge beam of the
steel frame and delivered to the braced frames.
Tributary weight of metal panel walls at braced frame bays = 1.6k
Tributary weight of panel walls between doors = 1.2k
Weight of all 12 metal roll-up doors = 14.4k
Total = 1.6k + 1.2k + 14.4k = 17.2 kips
Seismic Force = (0.12)(17.2) = 2.06 kips
of the longitudinal shear force goes to each braced frame bay = (2.06) = 0.515 kips
Total shear force = (5.09k + 0.515k) = 5.61 kips (24.95 KN)
1 kip = 4.448 KN
H1-17


 Previous Page
Previous Page
