5-3. Direction of soil movement
without the horizontal restraint on soil swell that
exists in the field and during one-dimensional consoli-
The foundation soil may expand both vertically and
dometer swell tests.
laterally. The vertical movement is usually of primary
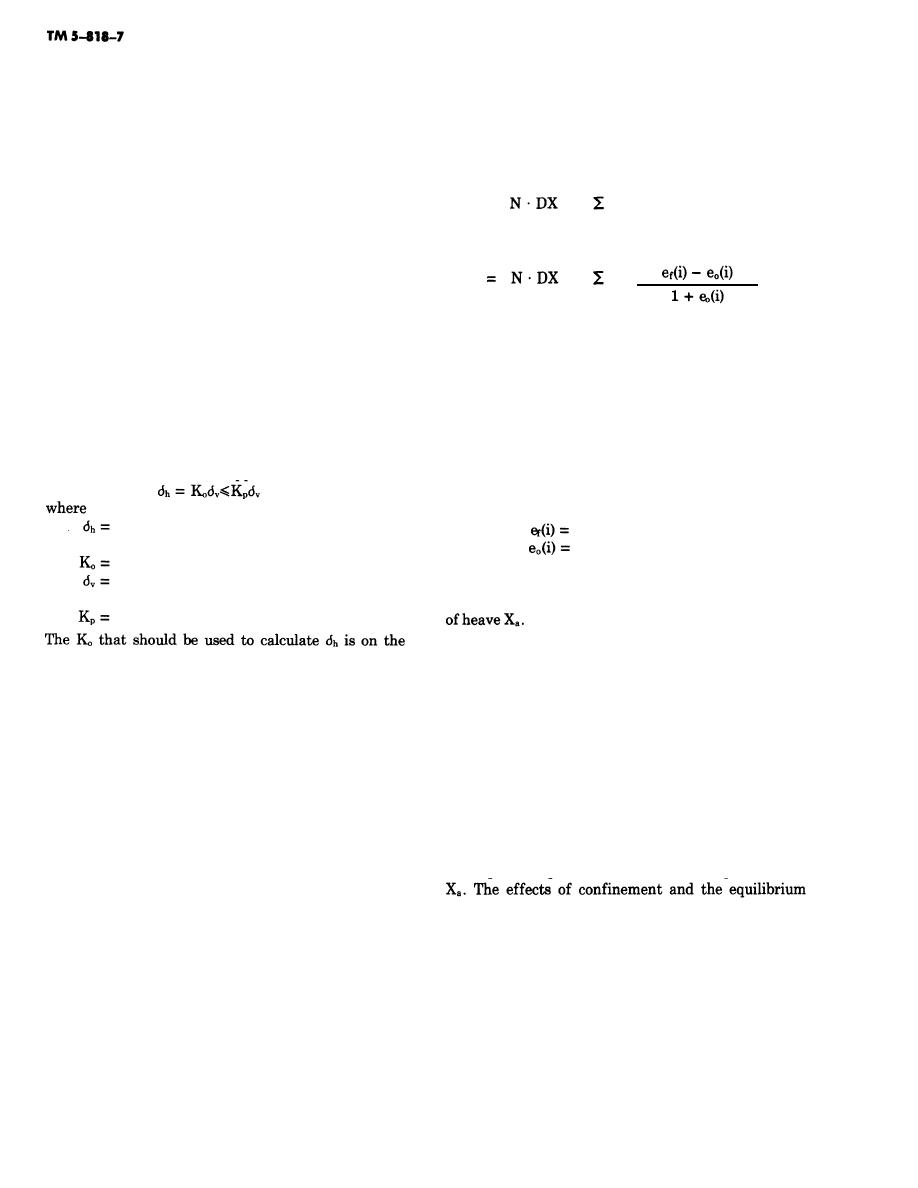
a. Basis of calculation. The potential total vertical --
interest, for it is the differential vertical movement
heave at the bottom of the foundation, as shown in fig-
that causes most damages to overlying structures.
ure 5-1, is determined by
a. Vertical movement. Methodology for prediction
i= NEL
of the potential total vertical heave requires an as-
DELTA(i)
AH=
sumption of the amount of volume change that occurs
i= NBX
in the vertical direction. The fraction of volumetric
swell N that occurs as heave in the vertical direction
i= NEL
depends on the soil fabric and anisotropy. Vertical
(5-2)
heave of intact soil with few fissures may account for
i= NBX
all of the volumetric swell such that N = 1, while
where
vertical heave of heavily fissured and isotropic soil
A H = potential vertical heave at the
may be as low as N = 1/3 of the volumetric swell.
bottom of the foundation, feet
b. Lateral movement. Lateral movement is very im-
N = fraction of volumetric swell that
portant in the design of basements and retaining
occurs as heave in the vertical di-
walls. The problem of lateral expansion against base-
rection
ment walls is best managed by minimizing soil volume
DX = increment of depth, feet
change using procedures described in chapter 7. Other-
NEL = total number of elements
wise, the basement wall should be designed to resist
NBX = number of nodal point at bottom
lateral earth pressures that approach those given by
of the foundation
(5-1)
DELTA(i) = potential volumetric swell of soil
element i, fraction
horizontal earth pressure, tons per square
final void ratio of element i
root
initial void ratio of element i
lateral coefficient of earth pressure at rest
The AH is the potential vertical heave beneath a flex-
soil vertical or overburden pressure, tons
ible, unrestrained foundation. The bottom nodal point
per square foot
NNP = NEL + 1, and it is often set at the active depth
coefficient of passive earth pressure
(1) The initial void ratio, which depends on geo-
logic and stress history (e.g., maximum past pressure),
order of 1 to 2 in expansive soils and often no greater
the soil properties, and environmental conditions
than 1.3 to 1.6.
shown in table 5-1 may be measured on undisturbed
5-4. potential total vertical heave
specimens using standard laboratory test procedures.
It may also be measured during the laboratory swell
Although considerable effort has been made to develop
tests as described in EM 1110-2-1906. The final void
methodology for reliable predictions within 20 percent
ratio depends on changes in the foundation conditions
of the maximum in situ heave, this degree of accuracy
caused by construction of the structure.
will probably not be consistently demonstrated, par-
(2) The effects of the field conditions listed in ta-
ticularly in previously undeveloped and untested
ble 5-1 may be roughly simulated by a confinement
areas. A desirable reliability is that the predicted po-
pressure due to soil and structural loads and an as-
tential total vertical heave should not be less than 80
sumption of a particular final or equilibrium pore
percent of the maximum in situ heave that will even-
water pressure profile within an active depth of heave
tually occur but should not exceed the maximum in
situ heave by more than 20 to 50 percent. Useful pre-
pore water pressure profiles are related to the final
dictions of heave of this reliability can often be ap-
void ratio by physical models. Two models based on re-
proached and can bound the in situ maximum levels of
sults of consolidometer swell and soil suction tests are
heave using the results of both consolidometer swell
used in this manual (para 4-2a).
and soil suction tests described in paragraph 4-2a. The
fraction N (para 5-3a) should be 1 for consolidometer
b. Pore water pressure profiles. The magnitude of
swell test results and a minimum of 1/3 for soil suction
swelling in expansive clay foundation soils depends on
test results. The soil suction tests tend to provide an
the magnitude of change from the initial to the equi-
upper estimate of the maximum in situ heave (N = 1)
librium or final pore water pressure profile that will be
in part because the soil suction tests are performed
observed to take place in a foundation soil because of
5-2


 Previous Page
Previous Page
